Every student at some point needs to master division using one or more of the various division strategies. One of the most effective and visual methods is the area model. This article explores the area model division method, why it’s the best method for most students and resources for mastering this method.
Division Strategies
Before diving deep into area model division, let’s touch on the other division strategies students might encounter.
These include partial quotients, long division, and the box method.
Each method has its own unique approach and benefits, but today, we’ll focus on the area model for division.

Enter your NON-WORK EMAIL to download freebies from my Freebie Library.
What is Area Model Division?
So, what exactly is area model division?
Picture a large rectangle representing the total value being divided.
This rectangle is then divided into smaller sections, each representing a part of the division problem.
By visualizing the problem this way, students can see how division works in a more concrete and understandable manner.

Why Use Area Model Division?
The area model division method is particularly beneficial for several reasons.
First, it helps students visualize the division process, making abstract concepts more concrete.
Second, it reinforces their understanding of place value and multiplication.
Finally, it breaks down complex problems into smaller, more manageable parts, building student confidence and competence.
Area Model Division Worksheets

Are you teaching area model division?
Do you need worksheets to review with your students or to check their understanding of division with and without remainders involving 1-digit and 2-digit divisors?
If so, these area model division worksheets are perfect for you.
They can be used for morning work, homework, warm-ups, and more.
2-Digit by 1-Digit Area Model Division

Let’s start with 2-digit by 1-digit area model division.
This involves dividing a 2-digit number by a 1-digit number using the area model approach.
To help your students master 2-digit by 1-digit area model division, we have some great worksheets.
These worksheets consist of 12 student pages with or without the 4.NBT.B.6 standard listed.
The worksheets feature multiple-choice and open-ended questions and are differentiated into two levels.
Level 1 has numbers 2-5 as divisors, while Level 2 has numbers 6-9 as divisors.
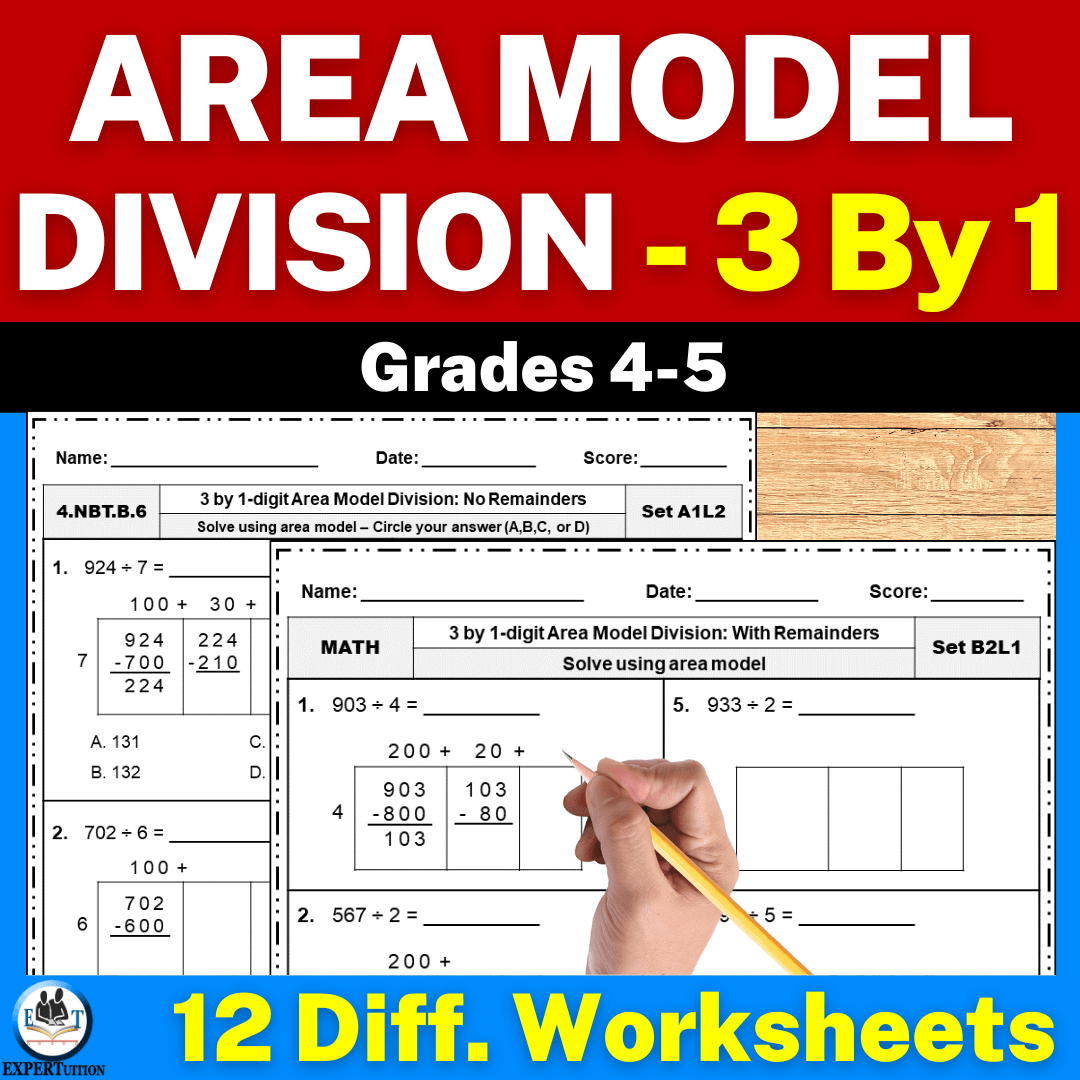
3-Digit by 1-Digit Area Model Division

Next, we move on to 3-digit by 1-digit area model division.
This is a bit more challenging but follows the same basic principles.
Students divide a 3-digit number by a 1-digit number using the area model.
Practice makes perfect, and our worksheets for 3-digit by 1-digit area model division are designed to help.
These worksheets also consist of 12 student pages with or without the 4.NBT.B.6 standard listed.
They feature multiple-choice and open-ended questions and are differentiated into two levels.
Level 1 has numbers 2-5 as divisors while level 2 has numbers 6-9 as divisors.
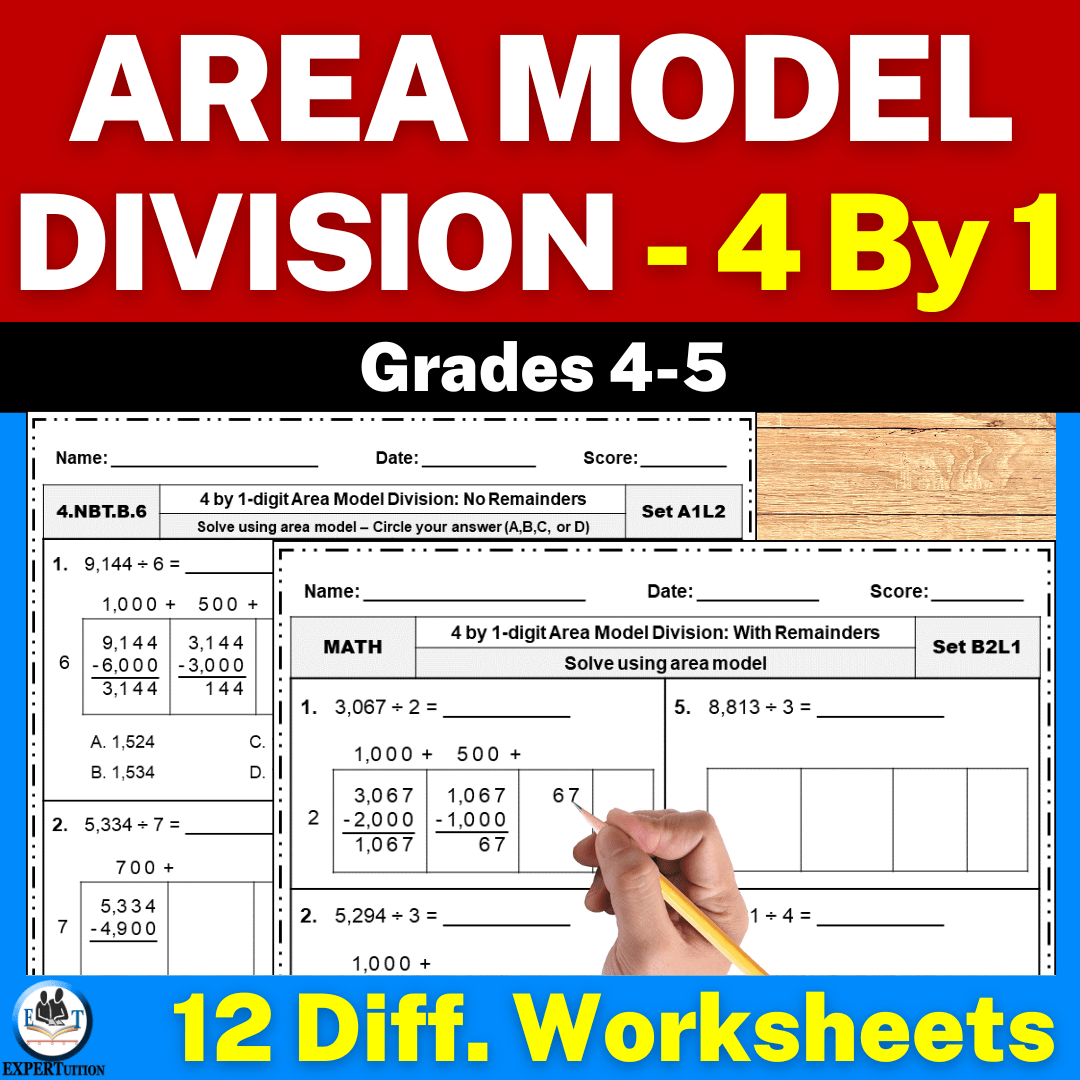
4-Digit by 1-Digit Area Model Division

As students progress, they can tackle 4-digit by 1-digit area model division.
This involves dividing a 4-digit number by a 1-digit number, using the same visual approach.
Our worksheets for 4-digit by 1-digit area model division are perfect for advanced practice.
These consist of 12 worksheets with or without the 4.NBT.B.6 standard listed.
The worksheets include multiple-choice and open-ended questions and are differentiated into two levels.
Level 1 features numbers 2-5 as divisors, while Level 2 includes numbers 6-9 as divisors.
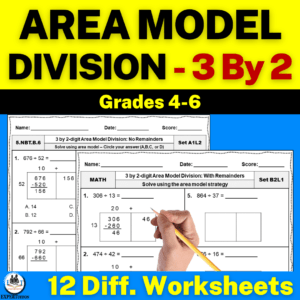
3-Digit by 2-Digit Area Model Division

Now, let’s step up the challenge with 3-digit by 2-digit area model division.
This involves dividing a 3-digit number by a 2-digit number using the area model.
Our worksheets for 3-digit by 2-digit area model division are designed to build confidence and competence.
These consist of 12 worksheets with or without the 5.NBT.B.6 standard listed.
The worksheets feature multiple-choice and open-ended questions and are differentiated into two levels.
Level 1 includes numbers 10-49 as divisors, while Level 2 includes numbers 50-99 as divisors.
4-Digit by 2-Digit Area Model Division

Finally, we reach the pinnacle: 4-digit by 2-digit area model division.
With this, students divide a 4-digit number by a 2-digit number using the area model.
Our differentiated worksheets for 4-digit by 2-digit area model division ensure every student can succeed.
These consist of 12 worksheets with or without the 5.NBT.B.6 standard listed.
The worksheets include multiple-choice and open-ended questions and are differentiated into two levels.
Level 1 features numbers 10-49 as divisors, while Level 2 includes numbers 50-99 as divisors.
Save with Bundles
You can save up to 25% when you purchase one of these bundles.



Why Use Worksheets?
Worksheets play a crucial role in mastering area model division.
They provide structured practice, allowing students to apply what they’ve learned in a controlled setting.
Whether it’s for morning work, homework, or warm-ups, these worksheets are invaluable.
Making Math Fun
One of the best parts of using area model division is that it makes math fun.
Incorporate fun activities into your lessons by creating division challenges or competitions.
By turning complex problems into visual puzzles, students can stay engaged and motivated.
They can see their progress and feel a sense of accomplishment as they tackle each part of the problem.

Enter your NON-WORK EMAIL to download freebies from my Freebie Library.
Conclusion
Incorporating area model division into your teaching toolkit can transform the way your students approach division.
It’s a powerful method that makes math accessible, engaging, and even fun.
With the right practice and support, your students will master area model division and build a strong foundation for future math success.
So grab those worksheets and get started on this exciting math adventure with your students!
You may also like these posts: